Casting Geometry, material and process determine the solidification time of a casting. The rudimentary equations that are required to estimate the casting solidification will be reviewed in this section. The occurrence of solidification shrinkage defect, which is indicated by the relationship between temperature, gradient and cooling rate, would also be looked at.
In order to derive the equation that would represent the solidification time of the simply shaped casting, the assumptions made are:
- The mold is made semi-infinite (the effect which the finite thickness of the mold has must be neglected), and the heat flow is unidirectional.
- Over a range of considered temperature the properties of metal and mold material are uniform (throughout the bulk), and remain constant.
- The mold surface and the metal are in complete contact (there are no air gaps).
- From the commencement to the end of solidification the metal-mold interface temperature remains constant.
An equation between the heat that the casting gives up Qcast, and the heat that the mould transferees Qmould, can give the solidification time. Here the casting volume (representing the heat content) is represented by V and the cooling surface area (through which heat is extracted), is represented by the A. The casting modulus is given by the ratio V/A. If two distinct shapes like a cube and a plate are considered to be having the same volumes then the shape whose cooling surface area is larger will be the first to be solidified.
If two or more than two castings that are simply shaped but have distinct volume and surface area of cooling are to be compared then the chvorinov's equation can be considered. If the solidification order of distinct regions of a casting is to be determined then the same chvorinov's principle can be used. This can be accomplished by dividing the casting into simpler shapes and then calculating the volume and surface area of cooling for every region. The hot spot is considered to be that region which has the maximum modulus and is the last to solidify.
The designing of the feeder is done in such a way that the modulus of the feeder is greater than the hot spot region's modulus. This criterion is the simplest for ensuring that the liquid state of the feeder is maintained till the feed metal is being supplied for the compensation of the casting's volumetric shrinkage.
Rate of Solidification: Using the following relation this can be determined in case of skin freezing alloys. If the casting that solidifies near a wall of mold with area A after an elapse of time , has a thickness d then we would have:
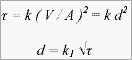
Earlier in 1935 researcher like Briggs verified experimentally the aforementioned relation by taking sets of castings and pouring them out after a time gap of different lengths. From 1940 to 1960, a great many researchers like Wlodawer, Pelliny, Ruddle, and Chvorinov verified the relation that exists between the solidification time and the casting modulus. Placing thermocouples in a mold and by obtaining cooling curves from each was the most widely used method.
- There is a limited application in practice of the equations between solidification time and rate. The casting's complex geometry, significance in the variation between mold and metal properties right from when it is poured to the solidus temperature, and also the effect that the variation in resistance has at the interface between the metal and the mould because of the air gap and the layer of oxide are mainly responsible for this.
- Attempts for the derivation of the equations that are improved by various researchers have met with limited success.
- The lack of correct thermal and physical data of varied castings and mold materials that are determined by the experiments are a further hindrance. At the solidification's end there is a requirement of the temperature's history given by T=T(x, y, z, t) for the regions while using Chvorinov's equation to determine the most probable regions of the shrinkage porosity.
- On the basis of this the peaks of temperature, rates of cooling, and gradients can be determined and thus the prediction of the shrinkage cavity's occurrence and its location can be made.
